NON-IONIZING ELECTROMAGNETIC RADIATION: TISSUE ABSORPTION AND SAFETY ISSUES
INTRODUCTION AND OBJECTIVES
An understanding of the interaction of electromagnetic radiation with tissue is important for many reasons, apart from its intrinsic interest. It underpins many imaging techniques, and it is essential to an understanding of the detection of electrical events within the body, and the effect of externally applied electric currents. In this text we assume that you have a basic understanding of electrostatics and electrodynamics, and we deal with the applications in other chapters. Our concern here is to provide the linking material between the underlying theory and the application, by concentrating on the relationship between electromagnetic fields and tissue. This is a complex subject, and our present state of knowledge is not sufficient for us to be able to provide a detailed model of the interaction with any specific tissue, even in the form of a statistical model.We have also limited the frequency range to <10 16="" frequencies="" higher="" hz="" i.e.="" ionizing="" p="" radiation="" radio="" to="" ultraviolet.="" waves="">Some of the questions we will consider are
Does tissue conduct electricity in the same way as a metal?
Does tissue have both resistive and capacitive components?
Do the electrical properties of tissue depend upon the frequency of electromagnetic radiation?
Can any relatively simple models be used to describe the electrical properties of tissue?
What biological effects might we predict will occur?
When you have finished this chapter, you should be aware of
The main biological effects of low-frequency electric fields.
How tissue electrical properties change with frequency.
The main biological effects of high-frequency fields such as IR and UV.
How surgical diathermy/electrosurgery works Some of the safety issues involved in the use of electromedical equipment.
There is some mathematics in this text, These two sections assume some knowledge of the theory of dielectrics. However, the rest should be understandable to all of our readers. The purpose of including the first two sections is to give a theoretical basis for understanding the rest of the text which covers the practical problems of tissue interactions with electromagnetic fields. If our understanding of these interactions is to be quantitative then we need to have a theoretical baseline.
TISSUE AS A LEAKY DIELECTRIC
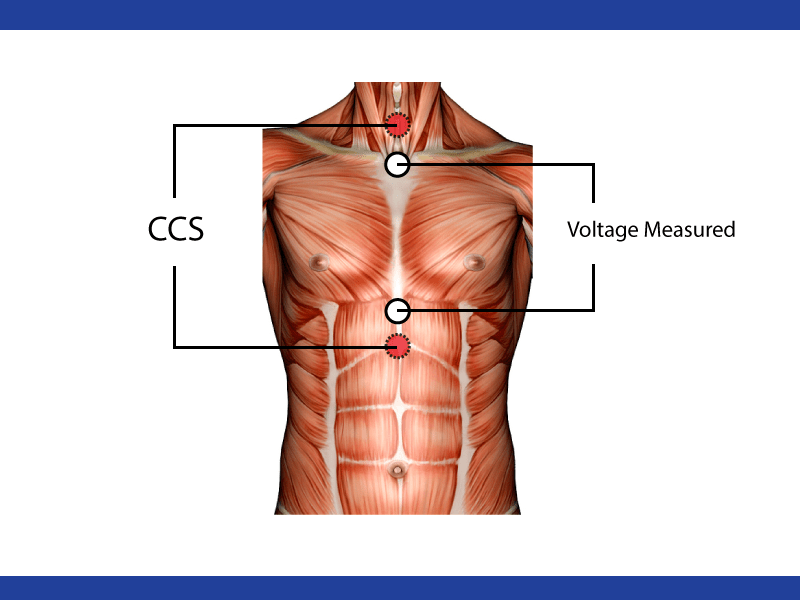
If two electrodes are placed over the abdomen and the electrical impedance is measured between them over a wide range of frequencies then the results obtained might be as shown in figure (1)
figure(1)
The results will depend somewhat upon the type and size of electrodes, particularly at the lowest frequencies, and exactly where the electrodes are placed. However, the result is mainly a function of the tissue properties. The impedance always drops with increasing frequency. This chapter is concerned first with trying to explain why tissue impedance changes with frequency in this way. It is an important question because unless we understand why tissue has characteristic electrical properties then we will not be able to understand how electromagnetic fields might affect us. We will start in this section by considering tissue as a lossy dielectric and then look at possible biological interactions with electromagnetic fields in later sections.
We are familiar with the concept of conductors, which have free charge carriers, and of insulators,
which have dielectric properties as a result of the movement of bound charges under the influence of an applied electric field. Common sense tells us that an insulator cannot also be a conductor. Tissues though contain both free and bound charges, and thus exhibit simultaneously the properties of a conductor and a dielectric. If we consider tissue as a conductor, we have to include a term in the conductivity to account for the redistribution of bound charges in the dielectric. Conversely, if we consider the tissue as a dielectric, we have to include a term in the permittivity to account for the movement of free charges. The two approaches must, of course, lead to identical results.
We will begin our exploration of the interaction between electromagnetic waves and tissue by exploring the properties of dielectrics. We are familiar with the use of dielectrics which are insulators in cables and electronic components. A primary requirement of these dielectrics is that their conductivity is very low (<10 alloys="" and="" by="" conduction="" conductivities="" electrons="" free="" have="" high="" in="" is="" m="" metals="" s="" the="" which="">10 4 S m −1 ). Intermediate between metals and insulators are semiconductors (conduction by excitation of holes and electrons) with conductivities in the range 10 0 –10 −4 S m −1 , and electrolytes (conduction by ions in solution) with conductivities of the order of 10 0 –10 2 S m −1 . Tissue can be considered as a collection of electrolytes contained within membranes of assorted dimensions. None of the constituents of tissue can be considered to have ‘pure’ resistance or capacitance—the two properties are inseparable.
We start by considering slabs of an ideal conductor and an ideal insulator, each with surface area A
and thickness x. If the dielectric has relative permitting ε r then the slab has a capacitance C = ε 0 ε r A/x. The conductance of the slab is G = σ A/x, where the conductivity is σ . It should be borne
Tissue with both capacitive and resistive properties in parallel. The capacitance and resistance
of the two arms are marked. in mind that the conductivity σ is the current density due to unit applied electric field (from J = σ E ), and the permittivity of free space ε 0 is the charge density due to unit electric field, from Gauss’ law. The relative permittivity ε r = C m /C 0 , where C 0 is the capacitance of a capacitor in vacuo, and C m is the capacitance with a dielectric completely occupying the region containing the electric field. This background material can be found in any book on electricity and magnetism. In tissue, both of these properties are present, so we take as a model a capacitor with a parallel conductance, The equations C = ε 0 ε r A/x and G = σ A/x define the static capacitance and conductance of the dielectric, i.e. the capacitance and conductance at zero frequency. If we apply an alternating voltage to our real dielectric, the current will lead the voltage.
Clearly, if G = 0, the phase angle θ = π/2, i.e. the current leads the voltage by π/2, as we would
expect for a pure capacitance. If C = 0, current and voltage are in phase, as expected for a pure resistance.
For our real dielectric, the admittance is given by Y ∗ = G + jωC, where the ∗ convention has been used to denote a complex variable (this usage is conventional in dielectric theory).
We can, as a matter of convenience, define a generalized permittivity ε ∗ = ε − jε which includes the effect of both the resistive and capacitive elements in our real dielectric. ε is the real part and ε is the imaginary part.
We can relate the generalized permittivity to the model of the real dielectric by considering the admittance,
Y ∗ = G + jωC = A (σ + jω ε 0 ε r ) x
EmoticonEmoticon