Particle in a One Dimensional Deep Potential Well
Let us consider a particle of mass ‘m’ in a deep well restricted to move
in a one dimension (say x). Let us assume that the particle is free inside the
well except during collision with walls from which it rebounds elastically.
The potential function is expressed as

for

……..(1.60)
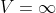
for

……..(1.61)
Figure 1.9 :
Particle in deep potential well
The probability of finding the particle outside the well is zero (i.e.
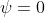
). Inside the well, the Schrödinger wave equation is
written as
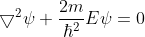
……..(1.62)
Substituting
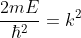
……..(1.63), and writing the SWE for 1-D
We
get
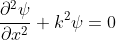
……..(1.64)
The
general equation of above equation may be expressed as
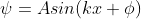
……..(1.65)
Where
A and
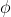
are constants to be
determined by boundary conditions
Condition
I: We
have ψ = 0 at x = 0,
therefore from equation (1.65)
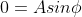
As
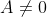
then
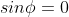
or
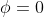
……..(1.66)
Condition
II: Further
ψ = 0 at x = L,
and
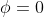
,therefore from equation
(1.65)
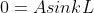
As
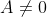
then
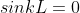
or

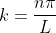
;
n = 1,2,3,4,…. ……..(1.67)
Substituting
the value of k from (1.67) to (1.63)
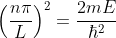
This
gives

 n = 1,2,3,4,…. ……..(1.68)
n = 1,2,3,4,…. ……..(1.68)
From
equation (1.68)
En is the
energy value (
Eigen Value) of the particle in a well. It is clear that
the
energy values of the particle in well
are discrete not continuous.
Figure 1.10 : Energy for
ParticleUsing
(1.66) and (1.67) equation (1.65) becomes, the corresponding wave functions
will be
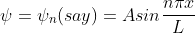
……..(1.69)
The
probability density


……..(1.70)
The probability density
is zero at x = 0 and x = L. since the particle is always within the well
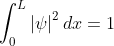




Substituting
A in equation (1.68), we get
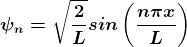
;
n = 1,2,3,4,…. …
(1.71)
The
above equation (1.71) is normalized wave function (
Eigen function)
belonging to energy value
En
Figure 1.11
: Wave function for Particle
EmoticonEmoticon